A 1.2 – Algebra Extension: the Meaning of Negative and Zero Exponents
Chapter 1, Section A2
Algebra Topics – Introduction to Zero and Negative Exponents; Combining Negative Numbers and Exponents
Elementary Education – Connections to Base Ten Decimals
Zero and Negative Exponents
Once again, we can let our experience in base ten help us discover what a zero exponent must equal and what negative exponents mean. Remember from the previous algebra section that we start base ten with the ones place and multiply by ten to get each next place value.
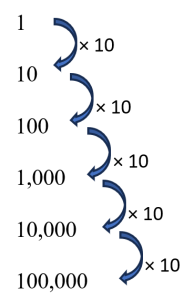
We can organize this into a table with each exponent.
| ? | 1 |
| 101 | 10 |
| 102 | 100 |
| 103 | 1,000 |
| 104 | 10,000 |
| 105 | 100,000 |
| 106 | 1,000,000 |
Zero Exponent – powers of ten
Using this table, what power of ten must logically go in that first row?
It must be 100. So that means that 100= 1. But why?
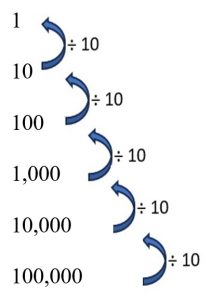
To find out, just as we multiplied by ten to go to the next higher power of ten, we should be able to go back from any power of ten to the previous power by dividing by ten.
We could start with 1,000,000 ÷ 10 = 100,000, going back one place value in the table.

Then we keep dividing by ten. 1,000 ÷ 10 = 100, dividing by ten again gives us ten, and then finally, we do the last division, 10 ÷ 10 = 1. Thus, we know that 100 = 1.
| 100 | 1 |
| 101 | 10 |
| 102 | 100 |
| 103 | 1,000 |
| 104 | 10,000 |
| 105 | 100,000 |
| 106 | 1,000,000 |
Zero Exponent – powers of two
Is it always true that a number to the zero power is 1? Let’s look at the powers of two for an additional example.
24 = 2 × 2 × 2 × 2 = 16.
If we divide by 2, we get what 23 must be, 8. And we know that’s true because 2 × 2 × 2 = 8
Divide by 2 again to get 4, which is 22, divide by 2 again to get 21=2, and finally, 2 ÷ 2 = 1, which must be 20.
So we know that 20 = 1.
Zero Exponent – powers of any expression
We can see logically that any number we use, when raised to the zero power, will give us 1. We can also use algebra to prove that anything to the zero power is 1.
We know that ![]() . We can also write this using exponents and use the rule that, when dividing variables with exponents, we subtract the exponents.
. We can also write this using exponents and use the rule that, when dividing variables with exponents, we subtract the exponents. ![]() . Since we can substitute any number, variable or expression in place of x, this proves that anything to the zero power is 1.
. Since we can substitute any number, variable or expression in place of x, this proves that anything to the zero power is 1.
Example 1 Simplify  .
.
Since the whole expression, ![]() , is raised to the zero power, the answer is simple:
, is raised to the zero power, the answer is simple: ![]() .
.
Example 2 Simplify 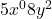 .
.
In this expression, only the x is raised to the zero power, so we replace only that part with 1. ![]()
Negative Exponents
The same base ten table we used at the beginning can be expanded to find the meaning of negative exponents.
| 10-3 | ? |
| 10-2 | ? |
| 10-1 | ? |
| 100 | 1 |
| 101 | 10 |
| 102 | 100 |
| 103 | 1,000 |
| 104 | 10,000 |
| 105 | 100,000 |
| 106 | 1,000,000 |
Fill in the missing values by dividing by 10. Start with 1 and divide by ten. It’s fine to use a calculator to do this. Try getting both the decimal and the fraction values (by changing your calculator settings).
Once you have your table filled in, look for patterns! What do you notice about the number of zeros in the fractions? How do they relate to the exponent?
| 10-3 | 0.001= |
| 10-2 | 0.01 = |
| 10-1 | 0.1 = |
| 100 | 1 |
| 101 | 10 |
| 102 | 100 |
| 103 | 1,000 |
| 104 | 10,000 |
| 105 | 100,000 |
| 106 | 1,000,000 |
Notice that ![]() ,
, ![]() , and
, and ![]() .
.
Another way to write this: ![]() ,
, ![]() , and
, and ![]() .
.
This leads us to the following rule of exponents: while positive exponents indicate repeated multiplication, negative exponents indicate repeated division. Written algebraically, we have ![]() .
.
Example 3 Rewrite  using only positive exponents.
using only positive exponents.
Since the whole expression has a negative exponent, this tells us that the whole expression gets divided. We have ![]()
Example 4 Rewrite  using only positive exponents.
using only positive exponents.
Since only the variable, x, is raised to the negative exponent, it is the only part that gets divided. We have ![]()
Combining Negative Numbers and Exponents
What is the difference between a negative number and a negative exponent? Recall that a negative number is a number to the left of zero on the number line — you can think of -3 as telling you how many times you subtract, while a negative exponent, as we have learned, indicates repeated division.
What happens when we combine negative exponents and negative numbers? What would be the answer to ![]() ? To find out, let’s start a little more simply, with a negative number and a positive exponent:
? To find out, let’s start a little more simply, with a negative number and a positive exponent: ![]() . Use the calculator below to change the exponent (right and left arrows) and the number (up and down arrows) until you get
. Use the calculator below to change the exponent (right and left arrows) and the number (up and down arrows) until you get ![]() .
.
You should see two possible answers, depending on whether you meant to have parenthesis around the -3 or not.
![]() , but
, but ![]() . Why?
. Why?
![]() means we are repeating the whole -3 twice:
means we are repeating the whole -3 twice: ![]() . Since a negative times a negative is a positive, we get positive 9.
. Since a negative times a negative is a positive, we get positive 9.
On the other hand, ![]() means that only the 3 is repeated twice:
means that only the 3 is repeated twice: ![]() . Since a negative times a positive is a negative, we get negative 9.
. Since a negative times a positive is a negative, we get negative 9.
Now let’s go back to our original question, ![]() . First, try the calculator, above.
. First, try the calculator, above.
Now let’s see why you got that answer.
We know that the negative exponent means to divide, or written algebraically, ![]() .
.
Thus, we get: ![]() .
.
We already know that ![]() , so we get
, so we get ![]() . If you divide, 1 ÷ 9, you will get the repeating decimal 0.1111…., the same as what the calculator above gave you. Notice that we have a positive answer, but not because the negative number and negative exponent “cancel” each other, but because we multiplied a negative by a negative.
. If you divide, 1 ÷ 9, you will get the repeating decimal 0.1111…., the same as what the calculator above gave you. Notice that we have a positive answer, but not because the negative number and negative exponent “cancel” each other, but because we multiplied a negative by a negative.
Example 5 Find 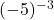 .
.
![]() . This is commonly rewritten as
. This is commonly rewritten as ![]() . Notice that now your answer is negative, since
. Notice that now your answer is negative, since ![]() .
.
Summary of Rules of Exponents
Here are the rules we discovered in this section and the previous one. Note that we have not yet practiced combining these rules, which we will do in a later section.
- Any expression (except for zero) to the zero power is 1. Written algebraically,
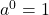 , where a ≠ 0.
, where a ≠ 0.
Tip: this includes the whole expression inside ( ). For example, ![]()
- Positive exponents indicate repeated multiplication. Written algebraically,
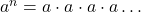 , where there are n factors of a.
, where there are n factors of a.
Tip: if we have an expression in parenthesis raised to an exponent, the whole expression is repeated, so any coefficient inside the parenthesis ends up raised to that power. For example, ![]() .
.
- Negative exponents indicate repeated division, Written algebraically, we have
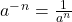 . If we have an expression in parenthesis raised to a negative exponent, the whole expression is divided.
. If we have an expression in parenthesis raised to a negative exponent, the whole expression is divided.
Tip: If only part of the expression is raised to a negative exponent, only that part is divided. For example, ![]() , but
, but ![]() .
.
- When multiplying exponential expressions that have the same base, add the exponents. Written algebraically, we have
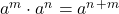 .
.
Tip: if there are coefficients in front of the variables, they still get multiplied. For example, ![]() .
.
- When dividing exponential expressions that have the same base, subtract the exponents. Written algebraically, we have
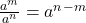 .
.
Tip: if there are coefficients in front of the variables, they still get divided. For example, ![]() .
.
- When we have an exponential expression raised to another exponent, we multiply the exponents. Written algebraically, we have
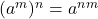 .
.
Tip: if there are coefficients in front of the variables, they still get raised to that exponent. For example, ![]()
- Negative exponents and negative numbers mean different things.
Tip: 4-1 is not -4, it is ![]() ; similarly, (-4)-1 is not 4, it is
; similarly, (-4)-1 is not 4, it is ![]() , commonly rewritten as
, commonly rewritten as ![]() .
.
Again, as you’re getting used to these rules, it’s always okay to expand the expression to see what answer makes the most sense! It is so important that math should make sense, and that you do not just blindly follow the rules someone told you.
Example 6 Which of the rules of exponents do we need to use to simplify 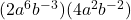 ? How can we write our answer using positive exponents only?
? How can we write our answer using positive exponents only?
We can look at the summary if we need help remembering which rule is which. Since our two expressions are next to each other, they are being multiplied, so we add the exponents — but the coefficients are still multiplied. ![]()
You may not remember how to find the answer to -3 + -2, so think about if you owe someone $3 and then you borrow another $2 from them. Now you owe $5, so -3 + -2 = -5. You might be thinking: “Wait, don’t two negatives make a positive?” That happens only with multiplication, not with addition.
We get ![]() . To write that using positive exponents only, we write
. To write that using positive exponents only, we write ![]()
Question: Now you try!
Example 7 What if we have a larger exponent in the denominator? How would we simplify  and write the answer using positive exponents only?
and write the answer using positive exponents only?
Notice that this example has a larger number and a larger exponent on the bottom, in the denominator. We can use the expanded version to check what to do.
![]()
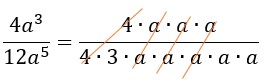
Now all of the variables on top, in the numerator, are “cancelled” since a/a = 1.
![]()
We can also work out the problem by subtracting the exponents. Since 3 – 5 = -2, we get ![]() . For the numbers, we could remember that while
. For the numbers, we could remember that while ![]() , if the larger number is on the bottom, we will still have a fraction:
, if the larger number is on the bottom, we will still have a fraction: ![]() . Done all together, we have
. Done all together, we have
![]()
Question: Now you try!
Video help can be found, here.
The homework below is a pdf of the online homework available in https://imathas.helpyourmath.com/. It does not include answers. Note that it is in two parts. In the first part, the homework contains images for each homework; the second part is text, to make the problems accessible to screen readers. Algebra extension homework A2
Unless otherwise specified, images in this section are by Kathleen Offenholley and licensed under a Creative Commons Attribution-NonCommercial 4.0 International License.